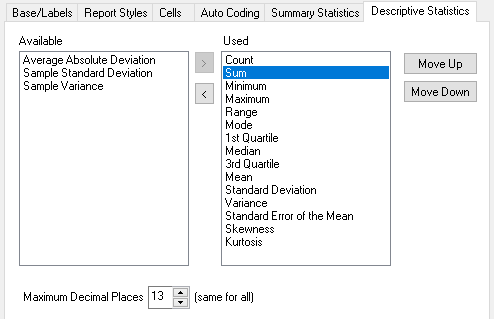
Descriptive Statistics tab

Statistic

Description

Count

The number of data cases

Mean

This is often called the average. It is defined as the sum of the items divided by the number of items. For example, for ten responses

Mean = (1 + 2 + 3 + 4 + 3 + 4 + 5 + 4 + 6 + 2) = 34 10 = 3.4

Mode

The mode of a distribution is the most frequent or most popular item. If two values tie for the mode, Snap chooses the lower. With the same ten responses: 1, 2, 2, 3, 3, 4, 4, 4, 5, 6

Mode = 4, since 4 is the most frequently occurring value (three occurrences).

Quartile 1

25% through a range of values

Median

The midpoint or 50% through a range of values. To calculate the median, the items of the distribution are arranged in order of magnitude starting with either the smallest or the largest, then:

if the number of items is odd, the median is the value of the middle item.

if the number of items is even, the median is the mean of the two middle items.

1, 2, 2, 3, 3, 4, 4, 4, 5, 6

Median = (3 + 4) ÷ 2 = 3.5

Quartile 3

75% through a range of values.

Sum

The sum is calculated by adding all the values of a distribution.

Sum = 1 + 2 + 3 + 4 + 3 + 4 + 5 + 4 + 6 + 2 = 34

Minimum

The minimum is the smallest value of the distribution.

Minimum = 1

Maximum

The maximum is the largest value of the distribution.

Maximum = 6

Range

The range shows the spread of the distribution and is calculated by subtracting the smallest value (minimum) from the largest value (maximum).

Range = 6 – 1 = 5

Standard Deviation

The standard deviation is a measure of dispersion of values in a distribution. It gives an indication of how much the values deviate from the mean. Thus, a distribution with a large range would have a larger standard deviation than one with a small range. The standard deviation is calculated as: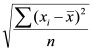

where x_i is each value in the distribution, is the mean of the values and n is the number of cases. For the sample in question:

Standard Deviation = 1.428286

Variance

The variance is another measure of dispersion of values in a distribution and is used in the calculation of the standard deviation:

Snap calculates the standard deviation and variance by assuming the data represents a sample rather than an entire population.

Standard Error of the Mean

The standard error of the mean is calculated by dividing the standard deviation by the square root of the number of items in the sample. It is defined as the standard deviation of the distribution of the sample mean and gives an indication of how far individual scores deviate from the mean score shown. The larger the sample, and/or the closer the individual scores are to the mean score, the smaller the standard error.

Standard Error of the Mean = 1.428286 ÷ √10 = 0.451664

Skewness

A distribution that is not symmetrical but has more cases toward one end of the distribution than the other is called skewed.

The measures of central tendency (mean, mode and median) can vary considerably. If the mean is larger than the mid point of the range (the median) and the most frequently occurring value (the mode), the sample is said to be positively skewed.

If the mean is smaller than the mid point of the range (the median) and the most frequently occurring value (the mode), the sample is said to be negatively skewed.

A small skewness value (close to 0) indicates that the data is evenly distributed about the mean. With this type of distribution it would be expected that the values for mean, mode and median be similar. The skewness of the example is 0.098843 indicating a small positive skewness.

Kurtosis

Kurtosis also gives an indication of the shape of a distribution in the form of the extent to which, for a given standard deviation, the data clusters around a central point.

A positive value for kurtosis indicates a distribution that is more peaked than usual. A distribution of this type would typically have most of the values clustered around a central point.

A negative value for kurtosis indicates a flatter or more widely dispersed distribution. The kurtosis for the example is -0.75202

Average Absolute Deviation

The average of the absolute deviations. It is a and tends to ignore distant outliers. It is a summary statistic of statistical dispersion and would normally only be displayed if specifically requested

Sample Standard Deviation

An estimate of the population standard deviation based on the sample.

Sample Variance

An estimate of the population variance based on the sample.